Geo-2017-Construction11-SimilarRightTriangles
Objective:
Demonstrate & prove Einstein’s Lesser Theorem through measurement and proof.
Visual Proof: Demonstration of Einstein's Lesser Thereom
How does the diagram above demonstrate that the blue and brown triangles are similar to the large triangle and to each other? (Hint: Consider the angles.)
Constructing the Diagram:
1. 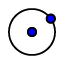 Construct a circle of any diameter.
2.
Construct a circle of any diameter.
2. 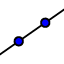 Construct a diameter using a line through the center and a point on the circumference.
3.
Construct a diameter using a line through the center and a point on the circumference.
3. 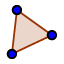 Using the ends of the diameter andany other point on the circle, construct a triangle.
4.
Using the ends of the diameter andany other point on the circle, construct a triangle.
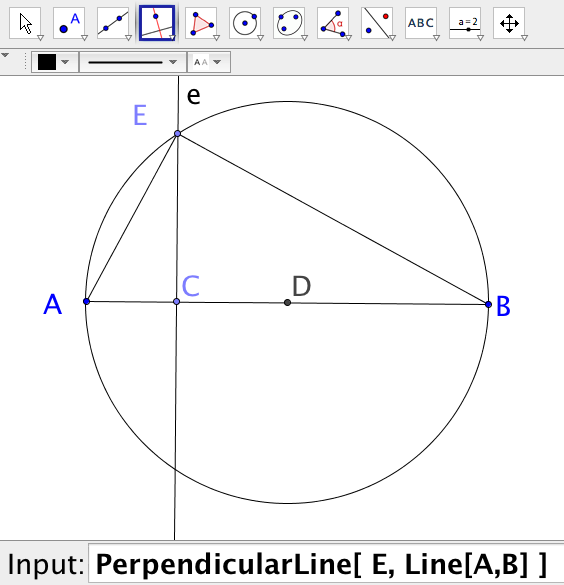
4. 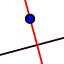 Construct the altitude of the triangle using the perpendicular line tool.
5.
Construct the altitude of the triangle using the perpendicular line tool.
5.  Label the intersection of the altitude and the base using the perpendicular line tool.
Label the intersection of the altitude and the base using the perpendicular line tool.
 Construct a circle of any diameter.
2.
Construct a circle of any diameter.
2.  Construct a diameter using a line through the center and a point on the circumference.
3.
Construct a diameter using a line through the center and a point on the circumference.
3.  Using the ends of the diameter andany other point on the circle, construct a triangle.
4.
Using the ends of the diameter andany other point on the circle, construct a triangle.
4.  Construct the altitude of the triangle using the perpendicular line tool.
5.
Construct the altitude of the triangle using the perpendicular line tool.
5.  Label the intersection of the altitude and the base using the perpendicular line tool.
Label the intersection of the altitude and the base using the perpendicular line tool.
Einstien's Lesser Theorem
Observation & Measurement
Measure the following using the measurement tool  or the
or the Length command.
CE by using Length[C,E]
AC by using Length[A,C]
CB by using Length[C,B]
Calculate the product of and record it below.
Calculate and record it on your diagram.
Paragraph Proof
Given: EC is the altitude of the right Prove: