Pythagorean Inequality Thm Activity
In the applet below, notice the white triangle with colored squares built off each side (orange, blue and pink).
Change the size and shape of this triangle by moving its BIG GRAY VERTICES around. For the moment, leave the red slider alone .
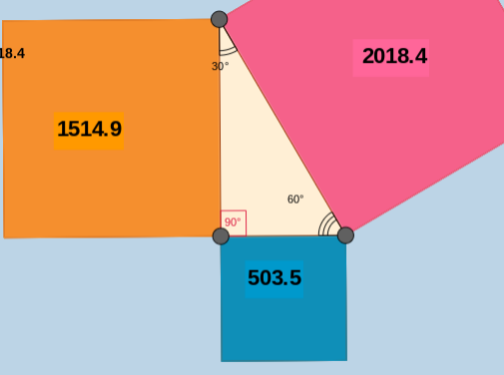
After interacting with the applet below for a few minutes, modify it until you get it as close as you can to the image below: 30-60-90 triangle; area of blue square around 500, area of orange around 1500, and area of pink around 2000 . Then follow the directions below.
ACTIVITIES:
#1.
With the angle opposite the pink square at 90 degrees, fill in line #1 of the worksheet. Compare the area of the biggest square to the sum of the two smaller squares.
#2.
Using the red slider, adjust the angle opposite the pink square to 120 degrees. Fill in line #2 of the worksheet and describe what has changed.
3.
Continue to increase the red slider so that angle opposite the pink square gets to 150 degrees and higher. Fill in line #3 of the worksheet and describe what has changed.
#4.
Reset the angle opposite the pink square to 90 degrees. Then using the red slider, adjust the angle down to 85 degrees, then 80 degrees, then 70 degrees. Continue to fill in the worksheet after each new angle.
Pythagorean Theorems
THEOREM 9.1__Pythagorean Theorem
In a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the legs.
THEOREM 9.2__ The Converse of the Pythagorean Theorem
If the square of the length of the longest side of a triangle is equal to the sum of the squares of the lengths of the other two sides, then the triangle is a right triangle.
THEOREM 9.3__ Pythagorean Inequalities Theorem
For any triangle ABC, where c is the length of the longest side, the following statements are true.
- If a2 + b2
<c2 , then triangle ABC is OBTUSE.
- If a2 + b2 = c2 , then triangle ABC is RIGHT.
- If a2 + b2 > c2 , then triangle ABC is ACUTE.
QUESTIONS
Question #1 Using your work from the activities above, explain Theorem 9.1 . Question #2 Using your work from the activities above, explain Theorem 9.2 . Question #3 Using your work from the activities above, explain Theorem 9.3 . Question #4 In several sentences, summarize your work, the Theorems, and what you have learn today.