Google Classroom
GeoGebraGeoGebra Classroom
GeoGebra

Introduction to Conic Sections

Author:Brenda Jiménez, carattinim, Irina Boyadzhiev

Relationships in Conic Sections

Relationships in Conic Sections
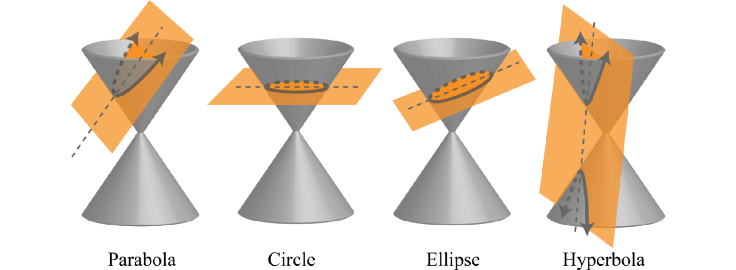
  • Conic sections can be seen as "slices" of two inverted cones. The shapes created by these "slices" are the same as the shapes which you will graph using equations.
  • The physical differences between sections are reflected in the equations of the sections.

Conics in General Form

Ax2 + Bx2 + Dx + Ey + F    Rules to Remember:
  • A and B cannot both equal zero - this would be the equation of a line
  • if A = B, the conic is a circle
  • if A or B = 0, the conic is a parabola
  • if A is not equal to B and AB > 0, the conic is an ellipse
  • if AB < 0, the conic is a hyperbola
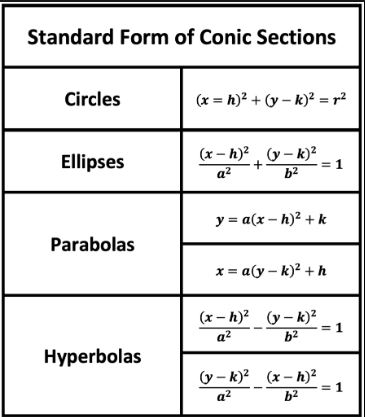
Image

Introduction to conics.

Use the graphic tool and classify the following conics.

(x+2)2+(y-3)2=4

Select all that apply
  • A
  • B
  • C
  • D
Check my answer (3)

What do you notice?

Is this relation in General form or Standard form?

Select all that apply
  • A
  • B
  • C
  • D
Check my answer (3)

What do you notice?

Is this relation in General form or Standard form?

Select all that apply
  • A
  • B
  • C
  • D
Check my answer (3)

What do you notice?

Is this relation in General form or Standard form?

Select all that apply
  • A
  • B
  • C
  • D
Check my answer (3)

What do you notice?

Is this relation in General form or Standard form?

Select all that apply
  • A
  • B
  • C
  • D
Check my answer (3)

What do you notice?

Is this relation in General form or Standard form?

Select all that apply
  • A
  • B
  • C
  • D
Check my answer (3)

What do you notice?

Is this relation in General form or Standard form?

Select all that apply
  • A
  • B
  • C
  • D
Check my answer (3)

What do you notice?

Is this relation in General form or Standard form?

New Resources

Discover Resources

Discover Topics