Google Classroom
GeoGebraGeoGebra Classroom
GeoGebra

Math 152 - Chapter 10 - Dynamic Worksheet

ForfatterTrevor Leach

Part A: Linear Angles

Use the following applet to answer question #1, #2, and #3 about Linear Angles

#1

Using the location of the two angles to write a definition for a linear angle pair.

#2

What do you observe about the angle measures of the linear angles?

#3

Make a conjecture about the sum of the measures of any linear angle pair.

Part B: Vertical Angles

Use the following applet to answer question #4 and #5 about Vertical Angles

#4

Based on the location of one of the vertical angle pairs above, write a definition for a vertical angle pair.

#5

Use points A, B, C, and D to change the angle values. Make a conjecture about the angle measures of a pair of vertical angles.

Part C: Additional Angle Pairs

Move the slider to the left so that it reads "Corresponding Angles." The color-coded angle pairs below are made when a transversal crosses two lines. These particular angle pairs are called corresponding angles.

#6

Move one of the lines by dragging one of the points on the line.  Notice that the pairs stay the same (the colors don’t change).  When do the angle pairs seem the most equal in size?

#7

Now, use the slider at the top of the screen to highlight some different angle pairs. (Not corresponding angles, but different angle pairs.)  Define these pairs based on their location with respect to the transversal.

  1. Alternate Interior Angles
  2. Alternate Exterior Angles
  3. Same Side Interior Angles
  4. Same Side Exterior Angles

Part D: The Parallel Postulate

Lines AB and FC are parallel. Line BC is called a transversal of lines AB and FC because it transverses (crosses) the lines.    Move the lines AB and FC by moving points A, B, or C.  Notice as you do, that the lines AB and FC remain parallel.  Answer the following questions.

#8

What are the colored angled pairs called?

#9

What do you notice about the angle measures of the angle pairs?

#10

 Fill in the blanks below to define the Parallel Postulate:  Parallel Postulate:  When two ________________________ lines are cut by a _________________________________ , the resulting __________________________________ angles are ______________________.

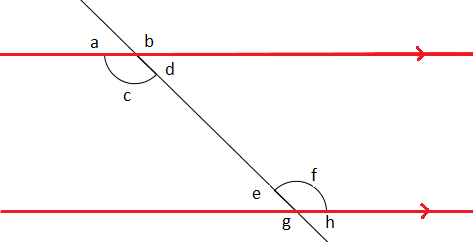
Image

#11

Use the Parallel Postulate to prove that if two parallel lines are cut by a transversal, then the alternate interior angles are congruent. (That is, c=f in the diagram above.)

Image

#12

Use the Parallel Postulate to prove that if two parallel lines are cut by a transversal, then same side interior angles are supplementary. (That is, c+e = 180 in the figure above.)

Nye Materialer

Opdag Ressourcer

Udforsk emner