Classwork #2.7 and #2.8 Summary
Video Summary
This video explains the main ideas from Classwork #2.7 and Classwork #2.8. Use the video to help you complete Homework #2.7 and Homework #2.8.
Classwork #2.7 Summary
Here are the prices for some smoothies at two different smoothie shops:

For Smoothie Shop A, smoothies cost $0.75 per ounce no matter which size we buy. There could be a proportional relationship between smoothie size and the price of the smoothie.
An equation representing this relationship is where represents size in ounces and represents price in dollars.
For Smoothie Shop B, the cost per ounce is different for each size. Here the relationship between smoothie size and price is definitely not proportional.
In grade 7, we do not have to write equations to relationship that are not proportional. You will do that next year in grade 8.
In general, two quantities in a proportional relationship will always have the same quotient. When we see some values for two related quantities in a table and we get the same quotient when we divide them, that means they might be in a proportional relationship—but if we can't see all of the possible pairs, we can't be completely sure. However, if we know the relationship can be represented by an equation is of the form , then we are sure it is proportional.
Classwork #2.8 Summary
If two quantities are in a proportional relationship, then their quotient is always the same. This table represents different values of and , two quantities that are in a proportional relationship.
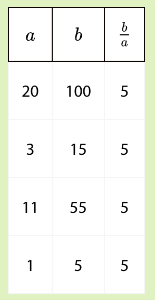
Notice that the quotient of and is always 5. To write this as an equation, we could say . If this is true, then . (This doesn’t work if , but it works otherwise.)
If quantity is proportional to quantity , we will always see this pattern: will always have the same value. This value is the constant of proportionality, which we often refer to as . We can represent this relationship with the equation (as long as is not 0) or .
Note that if an equation cannot be written in this form, then it does not represent a proportional relationship.