IM1H: 6.2 Leap Frog/Leap Year
Leap Frog
Use the check boxes and input boxes to transform one frog onto another.
- image 1 to image 2
- image 2 to image 3
- image 3 to image 4
- Which image was used to obtain image 5? How? Is it the only one?
Explain how you obtained each transformation.
Leap Year
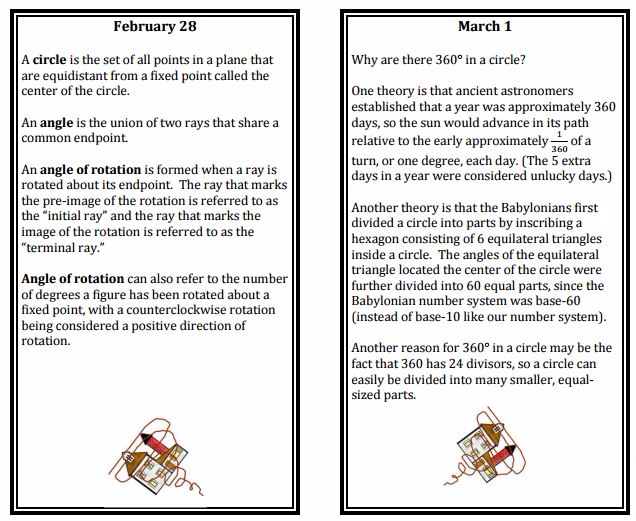
Carlos and Clarita are discussing their latest business venture with their friend Juanita. They have created a daily planner that is both educational and entertaining. The planner consists of a pad of 365 pages bound together, one page for each day of the year. The planner is entertaining since images along the bottom of the pages form a flip-book animation when thumbed through rapidly. The planner is educational since each page contains some interesting facts. Each month has a different theme, and the facts for the month have been written to fit the theme. For example, the theme for January is astronomy, the theme for February is mathematics and the theme for March is ancient civilizations. Carlos and Clarita have learned a lot from researching the facts they have included, and they have enjoyed creating the flip-book animation.
The twins are excited to share the prototype of their planner with Juanita before sending it to printing. Juanita, however, has a major concern. "Next year is a leap year," she explains, "you need 366 pages."
So now Carlos and Clarita have the dilemma of having to create an extra page to insert between February 28 and March 1. Here are the planner pages they ahve already designed.
Part 1
Since the theme for facts for February is mathematics, Clarita suggests that they write formal definitions of the three rigid-motion transformations they have been using to create the images for the flip-book animation.
How would you complete each of the following definitions? Use the following words and phrases in your definitions: Perpendicular bisector, center of rotations, equidistant, angle of rotation, concentric circles, parallel, image, pre-image, preserves distance and angle measures.
1. A translation of a set of points in a plane....
2. A rotations of a set of points in a plane...
3. A reflection of a set of points in a plane...
4. Translations, rotations and reflections are rigid motion transformations because...
Part 2
In addition to writing new facts for February 29, the twins also need to add another image in the middle of their flip-book animation. The animation sequence is of Dorothy's house spinning from the Wizard of Oz as it is being carried over the rainbow by a tornado. The house in the February 28 drawing has been rotated to create the house in the March 1 drawing. Carlos believes that he can get from the February 28 drawing to the March 1 drawing by reflecting the February 28 drawing, and then reflecting it again.
Using the image below, verify that the image Carlos inserted between the two images that appeared on February 28 and March 1 works as he intended.
Hint: To show the various reflections, create a polygon over the house on the main points, draw a "line" over the lines already created, and then use the reflection tool to check.
5. What convinces you that February 29 image is a reflection of the February 28 Image about the given line of reflection?
6. What convinces you that the March 1 image is a reflection of the February 29 image about the given line of reflection?
7. What convinces you that the two reflections together complete a rotation between February 28 and March 1 images?