da Vinci and others
Leonardo da VInci constructs an ellipse by projecting a semicircle upon an oblique line:
ambiguity too by Serlio
Not just Dürer is very ambigue by calling the ellips an eggline, but Serlio too is rather vague.
In De Geometria (Parijs 1545), the first of his 7 books on architecture Serlio writes about drawing special curves:
Vuole 'larchitetto fare un ponte o un arco, o anche una volta di minore altezza del mezzo erchio, cosa che molti muratori hanno certa pratica en col filo fanno grandi volto quali corrispondono all'ellisse en si raccordano con alcune ovale fatte col compasso.
"The architect wants to make a bridge or an arch, or a vault of lesser height of the half circle, which many bricklayers have some practice an with wire they make great vaults which corrispond to the ellipse.
They then connect them with some ovals made with a compass. "
Serlio refers to the rope method to draw ellipses but makes a difference between a theoretically correct line and building practice in which compassees are used to construct ovals. But he does not expose any of the properties of the ellipse. On the contrary he describes fout methods to construct ovals. In other words: ones had a basic theoretical knowledge of the ellipse. Tractates describe theoretial methods to construct ellipses point by point, but in building practice one used ovals.
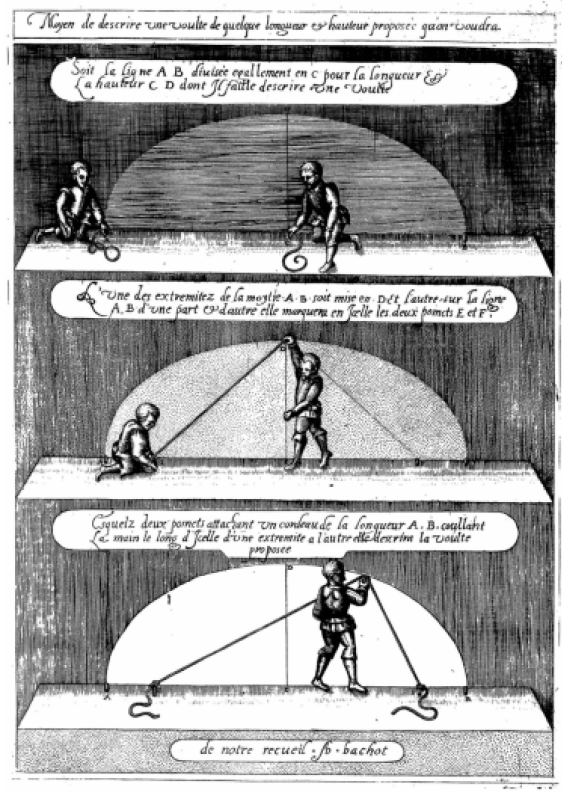
In Engeland the rope method to construct ellipses was called 'the gardener's method' since it was used to define flower beds.
In his book on architecture, fortifications an weapons of war Ambroise Bachot illustrates this method (1585).
confusion and ambiguity continuous...
Serlio en tijdgenoten kunnen we weinig verwijten. Van een vergelijking van de ellips was nog geen sprake en de analytische meetkunde werd pas geïntroduceerd in de 17e eeuw.
Maar ondanks de grote stappen die de wiskunde sindsdien zette, blijft de onduidelijkheid en de verwarring voortduren tot nu. In het artikel Ellipses and ovals in the physical space of St.Peter's square in Rome schrijven auteurs Alessandra Carlini en Paola Magrone het volgende:
"An ambiguity of use use between the terms ellipse and oval remains in some kind of literature, as toutistic literature and some school textbooks. For exemple, the Coliseum and the plan od St Peter's square are both described als elliptical shaped. Moreover, in thouristic guids (in the fout we consulted) St Peter's square is described al elliptical".
The autors also quote the bestseller book 'Angels and Demons' by Dan Brown:
"Two fountains flanked the obelisk in perfect symmetry. Art historians knew the fountains marked the exact geometric focal points of Bernini's elliptical piazza, but it was an architectural oddity Langdon had never really considered unti today. It seemed Rome was suddenly filled with ellipses, pyramids and startling geometry".
Further Serlio describes a second method. He writes:
"Nondimeno se l'architetto vorrà procedere teoricamente, portato dalla ragione, potrà tener questa via."
"Nevertheless, if the archtitect wants to try theoretically to be adresses by the reason it must take in account this method."
Follow the construction in the applet below.
- Draw two concentric half circles and devide them into 12 equal sectors.
- Draw vertical lines through the intersection points of the radii and the outer half circle.
- Draw horizontal lines from the intersection points of the radii with the inner half circle to the vertical lines of the according radii.
- Connect the intersection point with a continuous curve.